Solar Wind Clock Angle
Updates: every 10 minutes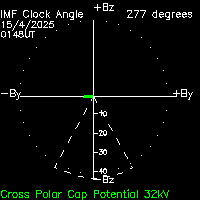
The solar wind interplanetary magnetic field is a three dimensional vector, "Bt", with components "Bx", "By" and "Bz". The vertical plane (to the ecliptic) is the "By", "Bz" plane, with "Bx" on the Sun-Earth line. When "Bz" has a negative orientation (ie "southward") the coupling to the Earths geomagnetic field is strongest. Southward interplanetary magnetic conditions are associated with geomagnetic storm activity. The 'clock angle' is the angle produced in the vertical plane from the vector addition of the "By" and "Bz" components of the interplanetary magnetic field.
Clock Angle in degrees: Angle = 0 : IMF Bz north Angle = 90 : IMF By +ve Angle = 180 : IMF Bz south Angle = 270 : IMF By -ve
The "clock hand" will change to red when the resultant vector is close to southward, and the "clock hand" is at least 15nT long. The plot below is of calculated solar wind clock angle and the ionospheric cross polar cap potentital, for a travelling 24 hour period, with the most recent data to the left. When the interplanetary magnetic field is very weak (i.e. the clock hand length is very small) the clock angle can become highly variable.
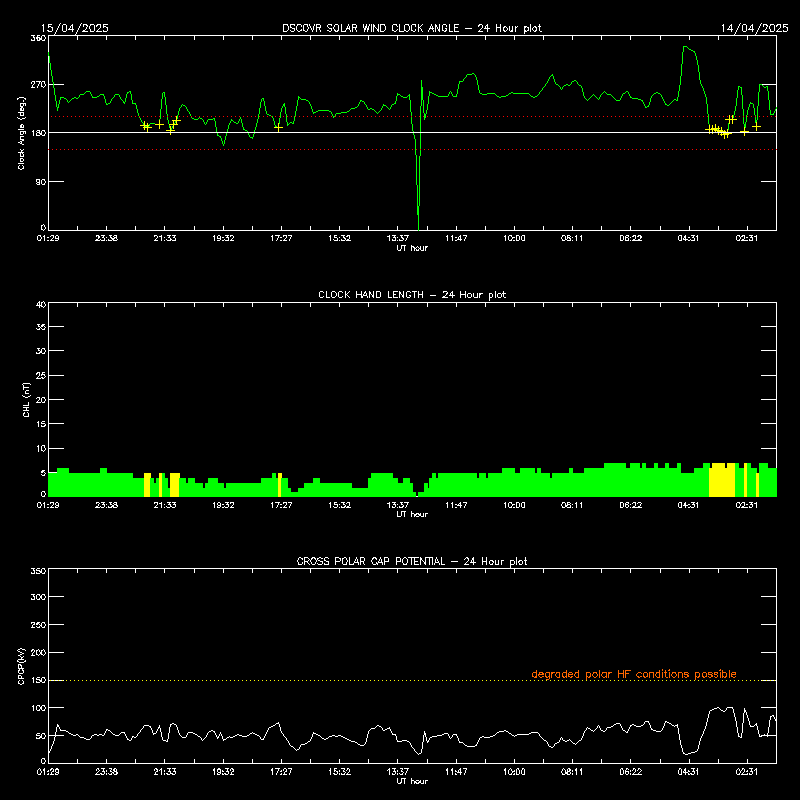
Colour of Solar wind clock angle within range of 150 to 210 degrees Data Point and the By-Bz plane IMF Clock Hand Length (CHL) Red CHL >= 15nT Orange 10nT >= CHL < 15nT Yellow 5 >= CHL < 10nT Green otherwise
Cross Polar Cap Potential and high latitude HF propagation
As the solar wind flows past the Earth's magnetosphere, an electric field is generated along the magnetopause in the dawn to dusk direction. The electric potential maps to the ionosphere in the open field line regions of the polar cap creating the cross-polar cap potential (CPCP). When the IMF turns southward and merging of the IMF with the Earth's magnetic field occurs, this electric field is enhanced. The dawn-to-dusk directed CPCP drives convection of ionospheric plasma in an antisunward direction across the polar cap and enhances the field-aligned closure currents (FAC) in the auroral zones. Hence higher solar wind speeds and stronger southward IMF enhance the polar cap convection patterns and the FAC in the auroral zones. Under conditions of enhanced convection and FAC additional energy is deposited into the auroral zones through direct particle precipitation and Joule heating causing a range of ionospheric effects that act to degrade or complicate conditions for HF communications.
Foremost amongst these is ionospheric heating that increases mixing in the thermosphere, the resulting composition changes leading to a reduction in the peak ionospheric densities and hence Maximum Useable Frequencies (MUFs) at high latitudes. The amount of energy deposited into the auroral zones in part dictates the extent of the equatorward extent of HF disturbance with larger events producing MUF depressions further equatorward. Secondary to the heating effects are the energetic particle precipitation effects. These are more sporadic and localised resulting primarily from substorm activity originating on the nightside. At the top end of the precipitation spectrum, energetic particles >10keV can cause D-region absorption of radio waves (Auroral Absorption or AA), particularly on the equatorward edge of the auroral zone around midnight and after local dawn. Statistically, AA peaks a few degrees equatorward of the visible auroral oval. D-region absorption attenuates low frequencies first and moves progressively to attenuation of higher frequencies with greater D-region ionisation. Complete absorption of radiowaves can occur. Less energetic particles (<10keV) can cause sporadic auroral-E (AE) layers to form at any point in the auroral oval which can screen radiowaves from the F layer and cause partial or complete propagation via the AE layer with unpredictable results. On the positive side, VHF communications which are normally restricted to line of sight propagation can range over very long baselines in the presence of AE layers that occur during enhanced activity. And during winter months it may well be the case that high latitude HF propagation is primarily supported by AE ionisation [Hunsucker and Bates, 1969]. Other HF propagation phenomena that can occur on high-latitude HF paths, particularly during times of enhanced activity are increases in Doppler shift and spread, fading and non-great-circle (NGC) propagation [Hunsucker and Hargreaves, 2003].
One might also expect enhanced cross polar cap flows during steady dayside reconnection (high CPCP). Whilst these antisunward flows of enhanced ionisation may well support frequencies well above the local polar cap background, they would also make HF propagation conditions less predictable in the polar cap region. The effect of the flows depends also on whether the HF propagation path has a reflection point near the flow axis (~00-12 MLT).
The CPCP is used here as a proxy for energy input into the ionosphere in the Polar Regions, primarily through the auroral zones with the assumption that this will in general degrade (or make less predictable) HF conditions in the Polar Regions via the above mechanisms. Whilst there are certainly some positive aspects of enhanced energy input into the polar ionosphere such as E-layer propagation, in general an increase in input energy will act to degrade or complicate HF conditions for propagation paths that intersect with the auroral and polar D-region or have E- or F-layer reflection points in or near the auroral zones.
The CPCP is sensitive to the orientation of the IMF and to the magnitude of the solar wind speed such that an increase in solar wind speed or a rotation of the IMF towards the southwards direction increases the cross-magnetospheric electric field and hence the CPCP. A number of empirical relationships between these parameters have been derived. Arguably the most robust of these was derived by Boyle et al (1997) through analysis of over three years of Defense Meteorological Satellite Program (DMSP) observations of CPCP and solar wind parameters. The empirical relationship is given by:
CPCP = 10E-4 Vsw^2 + 11.7 B (sin(theta/2))^3where:
CPCP is the ionospheric cross polar cap potential in kV
Vsw is the solar wind speed in km/s
B is the y-z plane component of the IMF field in nT
theta is the clock angle of the IMF in the y-z plane (0deg is northward and 180deg is southward)
The CPCP ranges from 10kV during slow solar wind and northward IMF to over 150kV during strongly southward IMF and high solar wind speeds.
References:
- Boyle, C. B., P. H. Reiff, and M. R. Hairston (1997), Empirical polar cap potentials, J. Geophys. Res., 102, 111
- Hunsucker, R.D and Bates, H.F (1969) Survey of polar and auroral region effects on HF propagation, Radio Sci. 4 347-365
- Hunsucker, R.D and Hargreaves, J.K (2003) The high-latitude ionosphere and its effects on radio propagation, Cambridge Univ. Press, UK
- Mike Terkildsen SWS Consultancy & Development 23 May 2007


